
The tesseract is mentioned in the children's fantasy novel A Wrinkle In Time, by Madeleine L'Engle, as a way of introducing the concept of higher dimensions, but the treatment is extremely vague. Tesseract projected onto a 3D plane, then projected onto a 2D plane.Ī hypercube is also used as the main deus ex machina of Robert J. In addition, a reference can be found in The Number of the Beast ( 1980), wherein the Burroughs continua device uses the hypercube principle to travel interdimensional universes to the incredible number of the beast. In Doctor Who, the main character pilots a time machine called a TARDIS, which is built with technology which makes it "dimensionally transcendental", that is, bigger inside than out.
4TH DIMENSIONAL HYPERCUBE SERIES
It is unclear if Glory Road was influenced by the debut of the science fiction television series Doctor Who on the BBC that same year. Glory Road ( 1963) included the foldbox, a hyperdimensional packing case that was bigger inside than outside. It collapsed, becoming a real hyperdimensional tesseract. an unfolding of the cells into three-dimensional space) of a tesseract. Robert Heinlein mentioned hypercubes in at least two of his science-fiction stories.And He Built a Crooked House ( 1940) described a house built as a net ( i.e. Tesseracts are also bipartite graphs, just as a path, rectangle, cube and tree are. The square, cube, and tesseract are all examples of measure polytopes in their respective dimensions. Thus the tesseract is given Schläfli symbol. The vertex figure of the tesseract is a regular tetrahedron. There are four cubes, six squares, and four edges meeting at every vertex. Three cubes and three squares intersect at each edge. Each pair of non-parallel hyperplanes intersects to form 24 square faces in a tesseract. This view is of interest when using tesseracts as the basis for a network topology to link multiple processors in parallel computing: the distance between two nodes is at most 4 and there are many different paths to allow weight balancing.Ī tesseract is bound by eight hyperplanes. The third diagram finally orders the vertices of the tesseract with respect to the distance along the edges, with respect to the bottom point. This picture also enables the human brain to find a multitude of cubes that are nicely interconnected. The second picture accounts for the fact that each edge of a tesseract is of the same length. The scheme is similar to the construction of a cube from two squares: juxtapose two copies of the lower dimensional cube and connect the corresponding vertices. The first illustration shows how a tesseract is in principle obtained by combining two cubes. In this fashion, one can obtain pictures that no longer reflect the spatial relationships within the tesseract, but which nicely illustrate the connection structure of the vertices. Furthermore, projections on the 2D-plane become more instructive by rearranging the positions of the projected vertices. This structure is not easily imagined but it is possible to project tesseracts into three or two dimensional spaces. Canonical coordinates for the vertices of a tesseract centered at the origin are (☑, ☑, ☑, ☑), while the interior of the same consists of all points ( x 0, x 1, x 2, x 3) with -1 < x i < 1.
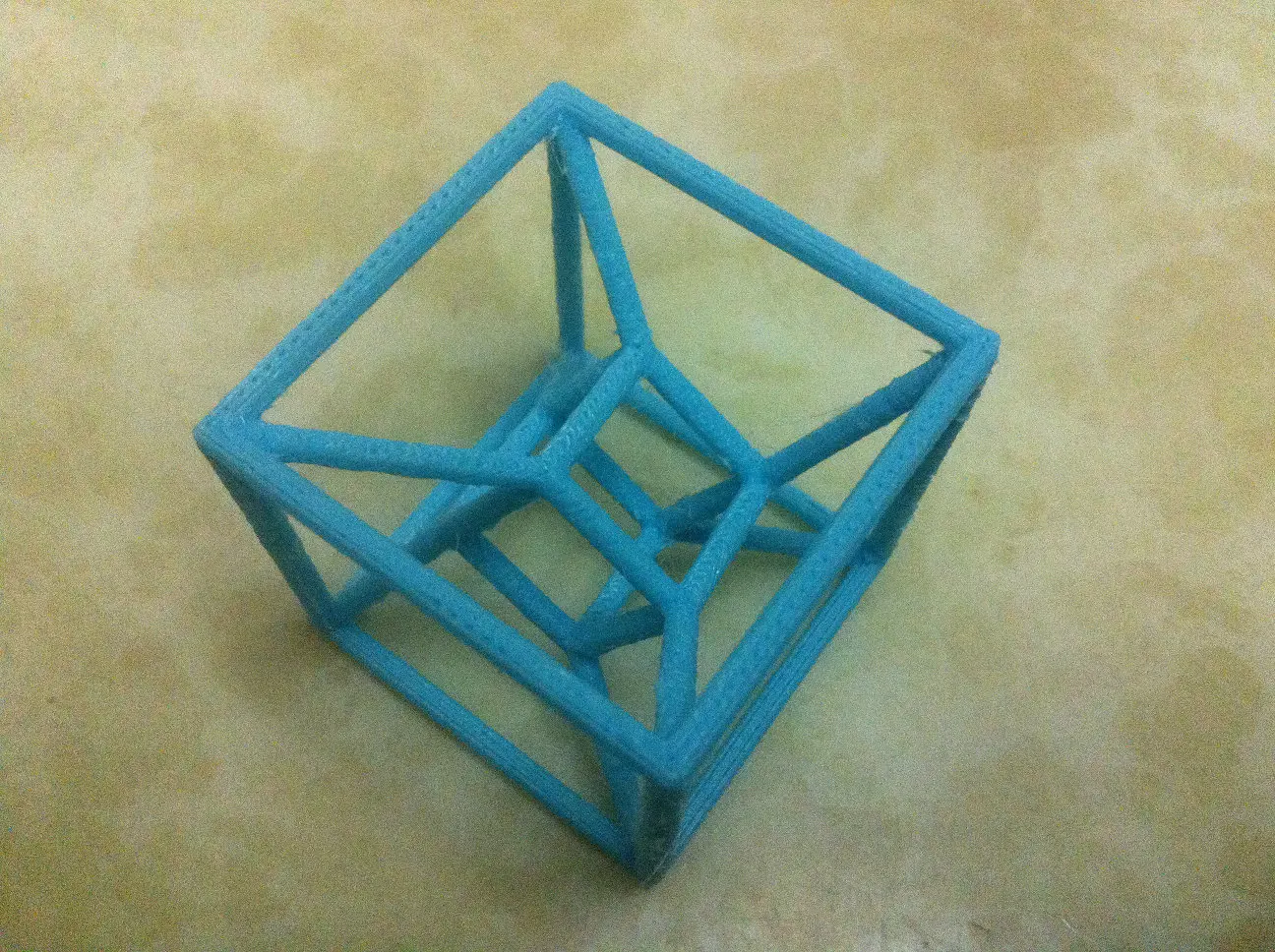
So this yields a total of 8 3-d faces.In a square, each vertex has two perpendicular edges incident to it, while a cube has three. However, we also get 3-d faces by taking any 2-d face (of which there are six, recall), and stretching it along the interval. So we get two 3-d faces by looking at $X \times \$, the two ends of the cube. As above, you can get a 4-cube $Y$ by starting with a 3-cube $X$ and "stretching it" along a unit interval $I = $. Since there are four of these, this yields four additional squares, for a total of six. However, we also have another source: Any edge of the original square, when we stretch it, becomes a new square itself. There are those that will be the ends of the extrusion, i.e. So where do we get each of the 2-dimensional faces from? Well, there are two types of 2-d faces that will arise. how we produce a cube by starting with a square, and "stretching" it along a unit interval in a perpendicular direction. Consider by analogy how we count the 2-dimensional faces of a cube, but based on looked at a cube as an "extruded" square, i.e.


 0 kommentar(er)
0 kommentar(er)
